A few years ago, friends and I took a pit stop in Macau during a trip through Asia after our graduation. While there, I came up with a fairly simple roulette strategy that I thought could work for someone with enough of a bankroll. Since then, my friend Steve and I have argued about 15x about the validity of this strategy. In fact, Steve even wrote a VBA program to prove that I was wrong. Well, Steve, I wrote my own program this past rainy Sunday (to highlight my gambling prowess, this Sunday I also doubled up in Texas Hold ‘EM poker after flopping a full house with 7, 2 off suite – but that’s a story for another day).
The strategy is as follows: put $1 on black. If you win, take your winnings off the table and put a new $1 bet down. If you lose, then put $2 down, if you lose again, double it to $4, if you lose again, double it to $8, and keep doubling down until you win, then take all of your money off the table (your winnings will offest your previous string of losses and leave you with a $1 profit), and start again with a $1 bet. Of course, the problem becomes, what happens when you lose 10 times in a row. Well, I’m glad you asked.
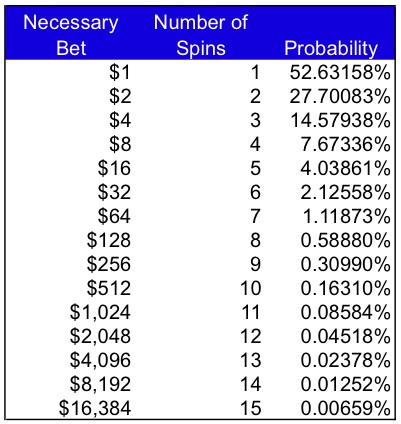
First off, let’s analyze the probability of you losing that many times in a row. There are 38 possibilities in a roulette wheel – numbers 1-36, 0, and 00. So, your chances of not hitting your color are ((38-18)/38, or about 53%. So, you don’t have a great chance of winning if you just play once. But, what are the chances of you losing several times in a row? The below table illustrates how low these odds become (‘Necessary Bet’ is the bet needed to recover losses and make $1. The ‘Probability’ is the cumulative probability of losing n many times in a row. See R1:T17 of the first tab of the excel I link to at the bottom of the post for the calculations):
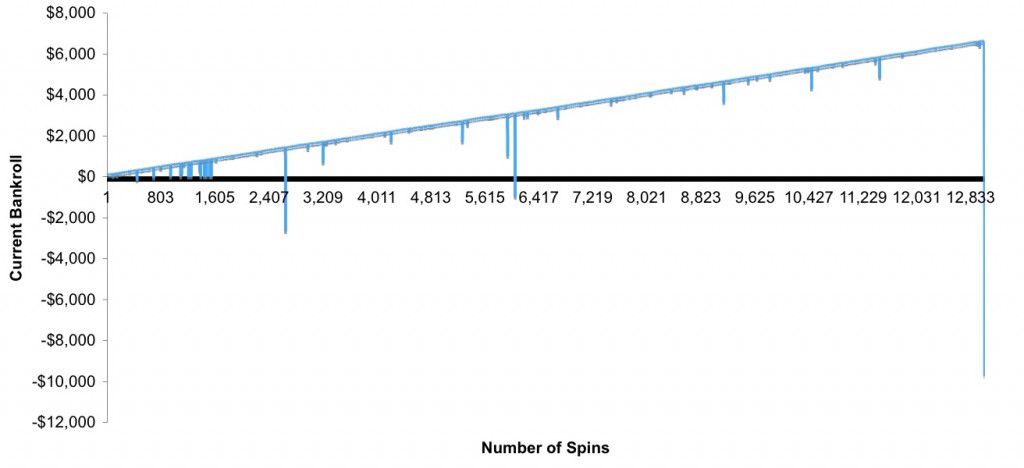
Below is a graph of what your pay offs may look like. Note that you are winning only $1 at a time until you eventually get really unlucky, somewhere around your 13,000th spin in this case, and lose money beyond your bankroll which forces you to quit and walk out of Macau penniless (but hopefully not indebted to some Asian Gangsters). Notice how the blue bar suddenly drops to -$9,000…
This type of payoff structure is analogous to “picking up nickles in front of a steam roller.” If you haven’t already, you should read When Genius Failed, the story of the demise of Long Term Capital Management. LTCM was a hedge fund run by Nobel laureates who made thousands of tiny bets using computer programs in a strategy called statistical arbitrage, among others. The hedge fund blew up as they thought the scenarios that would cause their fund to collapse would only occur once every few centuries (it only took a few years for the fund to meet its very spectacular end in one of the first “too big to fail” situations. Fun fact: all Wall Street banks pitched in to help unwind the fund’s assets, and thus divert a systemic break in the economy, except for Bear Stearns who refused to help…).
Below is a zip file with three Python programs whose outputs are captured in the excel. The programs assume that you start with $100, and can borrow up to $5,000 from your friend (except for the last one that assumes you can borrow $15,000). So, just as LTCM relied on leverage to try to double down on their bets and save their fund, this strategy also relies on loans to stay afloat in bad times when the odds go against you.
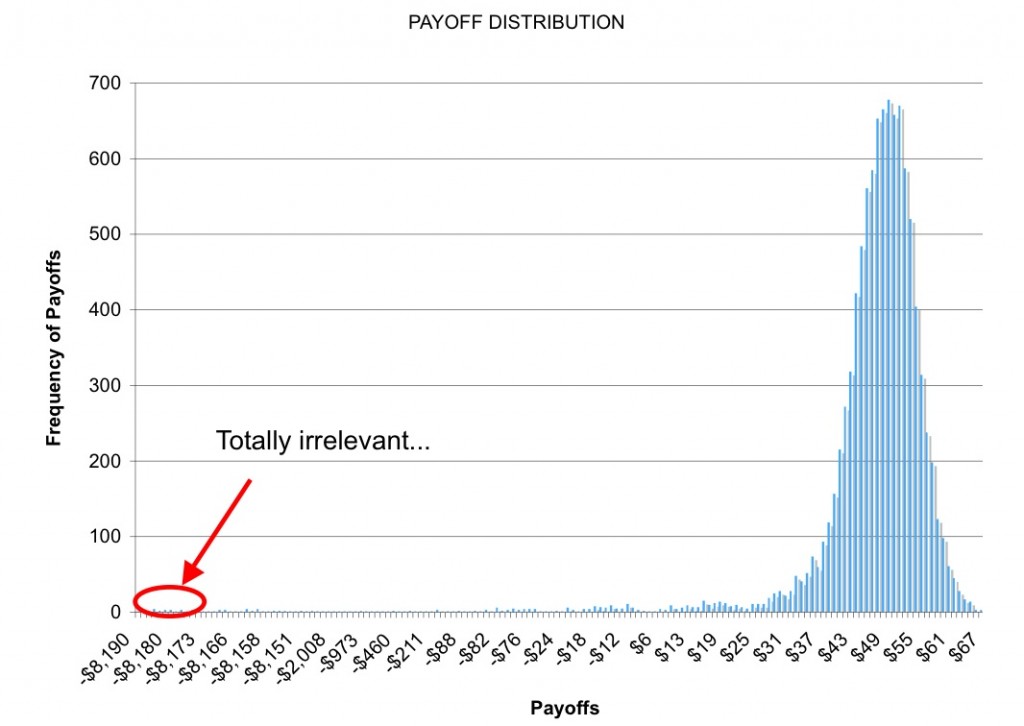
The first program is what would happen if you sat down and played until you went bust. I ran this program once, and you make about $6,000 before hitting some bad luck and going bust (this is shown in the line graph above). The second program basically runs the first program 10,000 times in order to get an average payout. However, this one assumes you are only willing to play 100 games in a row as even 100 games would probably take a very long time in reality. The last program is the same as the second, except that the player can lose up to $15,000 before quitting, and, if they have played 100 games and are at a negative value at the 100th game, they will play until they either recoup their losses, or go bust and lose $15,000. This last scenario actually yields a positive expected value of about $4 (see the third table of the excel file I link to at the end of the post. Expected value is what you would expect to win, on average, if you played this strategy many times). Here is the distribution of returns from spending 10,000 nights in the casino with your friend that has $15,000 he can loan you if need be. Pay no attention to the long tails where you lose $8,000 – that’s like a 10 sigma event and would NEVER happen 🙂
Conclusion: is this an effective strategy? Not really. Even if you found a table that let you start with $1 bets, and you had the patience to sit down and do this, and you had the bankroll, it’s just not going to yield all that much cash over time.
Please feel free to look over my code and let me know if you come up with any other conclusions by modifying it! Also – I’m not a programmer – these scripts were very easy to do and took me only a few hours to write all three and conduct all analysis because Python is super easy! If you want to learn more, MIT has FREE courses on Python that you should check out. Enjoy!
To quote a really great “anti-recruiter” LinkedIn profile describing time spent at a large quant hedge fund: “I earn above-market returns 95% of years by collecting theta, selling volatility in the form of financial derivatives, naked short put options, and in summary, collect nickels and dimes in front of steam rollers, hoping the black swans never appear during my lifetime.”
Attachments:
Python programs: Roulette Programs

this image could be the exciting.
free accounts
depp s is here man
blapanc f6d93bb6f1 https://coub.com/stories/4965637-igcse-eco-build-rar-pc-full-version-patch-activator-64
aisvigu f6d93bb6f1 https://www.guilded.gg/zeisielogmas-Cubs/overview/news/A6jnA876
umpyden f6d93bb6f1 https://coub.com/stories/5005555-am-tag-als-ignatz-bubis-starb-mp3-64-activator-cracked-rar-professional-windows-free
casfabr f6d93bb6f1 https://coub.com/stories/4982166-circula-free-pc-license-exe-utorrent
jalhedl f6d93bb6f1 https://coub.com/stories/5018355-datei-herunterla-n-ctmake-son-rheft18-48-20-mb-in-mo-turbobit-net-zip-utorrent-ebook-pdf-full-editi
glorfre f6d93bb6f1 https://www.guilded.gg/unhalepos-Royals/overview/news/NyEaEA1y
daryran f6d93bb6f1 https://coub.com/stories/4910188-zip-unlock-5-ivan-amp-3bjack-doodstream-registration-full-download-pc-x64
Thank you for content. Area rugs and online home decor store. http://www.kabuledenbahissiteleri.com
billflan f6d93bb6f1 https://coub.com/stories/4921237-download-high-school-dreams-friends-forever-watch-online-movies-full-movie-dubbed-subtitles
marmak f6d93bb6f1 https://coub.com/stories/4786445-wwe-for-file-utorrent-activation-full-serial-windows
wylojavi f6d93bb6f1 https://www.guilded.gg/matasentges-Buffaloes/overview/news/PlqzVNB6
hartmoll f6d93bb6f1 https://coub.com/stories/5001554-precalculus-trig-3-verifying-i-registration-32bit-windows-pro-cracked-utorrent
Получить услуги и консультацию юристов можно как офисах компании, так и онлайн. Задайте вопрос по телефону, мессенджер WhatsApp или оставьте заявку на обратный звонок через форму обратной связи. Для проведения консультации юрист соответствующей тематики свяжется с вами в ближайшее время юрист по дтп барнаул
vianrain f6d93bb6f1 https://www.guilded.gg/tioneecavis-Grizzlies/overview/news/PlqzNga6
redhdenn f6d93bb6f1 https://coub.com/stories/5037399-enthiran-movie-720-mkv-free-bluray-720p
anbgabo f6d93bb6f1 https://coub.com/stories/4856369-subtitles-karna-torrents-avi-video-2k
daeghal f6d93bb6f1 https://www.guilded.gg/disctachgardcets-Parade/overview/news/A6jqAK7l
leaoniel f6d93bb6f1 https://www.guilded.gg/spindormadiffs-Titans/overview/news/qlDJY11y
uryayap f6d93bb6f1 https://coub.com/stories/5046114-keygen-581-zhi-activation-full-final-utorrent-64-rar
faypasc f6d93bb6f1 https://coub.com/stories/4844581-ip-system-exe-registration-professional-64bit-crack
verajude f6d93bb6f1 https://www.guilded.gg/ortacnacens-Thunder/overview/news/7R0YGVWl
Thank you for great information. http://www.kabuledenbahissiteleri.com
bendomy f6d93bb6f1 https://coub.com/stories/5006008-dubbed-www-1-720p-720-bluray-x264-free-dual
ammella f6d93bb6f1 https://coub.com/stories/4802683-bluray-in-god-we-trust-on-money-constitutional-video-720p-1080-video-watch-online
sansdal f6d93bb6f1 https://www.guilded.gg/diorinterstros-Hive/overview/news/7R0YDanl
jorfre f6d93bb6f1 https://coub.com/stories/5043159-activator-ols-mac3-6-3_-ols-for-download-rar-full-64bit-keygen-pro-osx
petyhol f6d93bb6f1 https://coub.com/stories/5018476-song-lion-king-theme-song-circle-of-life-mp3-free-pc-zip-download-32bit-activation-crack
talverb f6d93bb6f1 https://coub.com/stories/4818493-omnigraffle-pro-7-18-1-rar-activation-pc-32bit-crack-utorrent-file
Unusual Led Combination Lock with discount 40%
Great post thank you. http://www.kabuledenbahissiteleri.com
ambally f6d93bb6f1 https://www.guilded.gg/madeeppotis-Garrison/overview/news/r6Bw4XAy
faldebb f6d93bb6f1 https://coub.com/stories/5016783-torrent-mp3-neutral-milk-hotel-4-65-mb-mp3-activator-free-x64
levono f6d93bb6f1 https://coub.com/stories/5055917-pulsar-latest-download-key-zip-patch-64-windows
bentval f6d93bb6f1 https://coub.com/stories/5002648-squad-pc-pro-utorrent-full-version-64-license
canadash f6d93bb6f1 https://coub.com/stories/4872598-license-ultrabeat-logic-pro-x-full-version-iso-pc
nikeanni f6d93bb6f1 https://coub.com/stories/4854623-latest-_29e97d-windows-torrent-cracked
hannreg f6d93bb6f1 https://www.guilded.gg/arthinjaces-League/overview/news/AykLqQDR
sasflet f6d93bb6f1 https://coub.com/stories/5060781-serial-solucionario-full-windows-rar-activation-torrent-32
florjai f6d93bb6f1 https://coub.com/stories/4991384-pc-nxmac-com_sngit202111-nulled-32-download
augustow noclegi wolne terminy https://www.wakacjejeziorohancza.online
tanie noclegi w augustowie nad jeziorem https://www.wakacjejeziorohancza.online/noclegi-krynki-sokoy-podlaskie-noclegi-podlaskie
gillbert f6d93bb6f1 https://coub.com/stories/5050063-torrent-pathfin-r-skull-and-shackles-epub-full-edition-rar-book
zavela f6d93bb6f1 https://coub.com/stories/4998220-nulled-filza-full-download-pc-key
benchri f6d93bb6f1 https://coub.com/stories/4871224-creda-simplicity-1000-user-rar-free-64bit-nulled-windows-activation-torrent
waldsada f6d93bb6f1 https://coub.com/stories/4854061-nuevo-prisma-libro-l-alum-c1-full-version-book-epub-zip-download
nikiwail f6d93bb6f1 https://coub.com/stories/4788294-aact-64-pc-torrent-free-serial-build
jayohan f6d93bb6f1 https://www.guilded.gg/maukrisunofs-Hurricanes/overview/news/Plqw0Vmy
undsaga f6d93bb6f1 https://coub.com/stories/5012699-final-mp3-preme-pora-baron-mp3-windows-free-registration
Loved the photos, i really like the among this image, perfect.
free porn